or: Why is the square of the nth triangular number equal to the sum of the first n cubes?
SUGGESTED LEVEL: Further Maths A-level or anyone at any level if you love numbers!
Every New Year brings a new number for us all to wonder about: is this year a prime number? A square number? Something else?? As I write we are at the start of 2025 and the interest has been far higher than usual. So: why is 2025 such a very cool number?
SHORT ANSWER (read on if this makes no sense – I will explain!):
$2025=(1+2+3+4+5+6+7+8+9)^2=1^3+2^3+3^3+4^3+5^3+6^3+7^3+8^3+9^3$.
You can easily use a calculator to check that it’s true, but my maths brain really wants an intuitive way to see why this works – ideally using geometry (“shapes”) so I can picture the result!
LONGER ANSWER: $2025$, being $45\times 45$, is what we call a square number i.e. a number multiplied by itself: $2025=45^2=45\times45$.
Not only that: the number 45 (that 2025 is the square of) is itself a triangular number i.e. a number which is the sum of the first few whole numbers: $45=1+2+3+4+5+6+7+8+9$.
Not only that – and here is the really cool bit – 2025 is also the sum of the first nine cube numbers (i.e. a number multiplied by itself three times).
If this all makes sense, feel free to jump to SO WHY DOES THIS HAPPEN. If not: read on!
WHY ALL THE SHAPES? This picture explains why triangular and square numbers are so-called: they count the numbers of blobs in each of these triangles and squares!

$1+2+3=4$ red blobs literally makes a triangle shape! And an array of $4^2=16$ blue blobs literally makes a square shape! In a similar way, if you had $4^3=4\times4\times4=64$ building blocks you could arrange them into a cube shape (four blocks wide, four deep and four tall – like a Rubik’s cube but with four along each edge instead of three). A short way of writing triangular numbers is $T_n$, so e.g. $T_5 = 1+2+3+4+5$.
IS 2025 UNIQUE IN HAVING THIS PROPERTY? No! Something similar happens whenever a year is the square of a triangular number. For instance:
$1=1^2=1^3$
$9=(1+2)^2=1^3+2^3$
$36=(1+2+3)^2=1^3+2^3+3^3$
$100=(1+2+3+4)^2=1^3+2^3+3^3+4^3$
The current year 2025 is the ninth row in this pattern (because it’s the square of 45 which is the ninth triangular number $T_{9}=45$). So the next time this will happen is in the year $(T_{10})^2=55^2=3025$.
SO WHY DOES THIS HAPPEN?
By which we mean: why is the square of the sum of the nth triangular number always equal to the sum of the first n cube numbers? For anyone taking a Further Maths A-level, the proof of this result is a standard exercise in proof by induction (a very sneaky and powerful algebraic technique), but for everyone else, here’s a way of thinking about it geometrically – so using shapes rather than algebra.
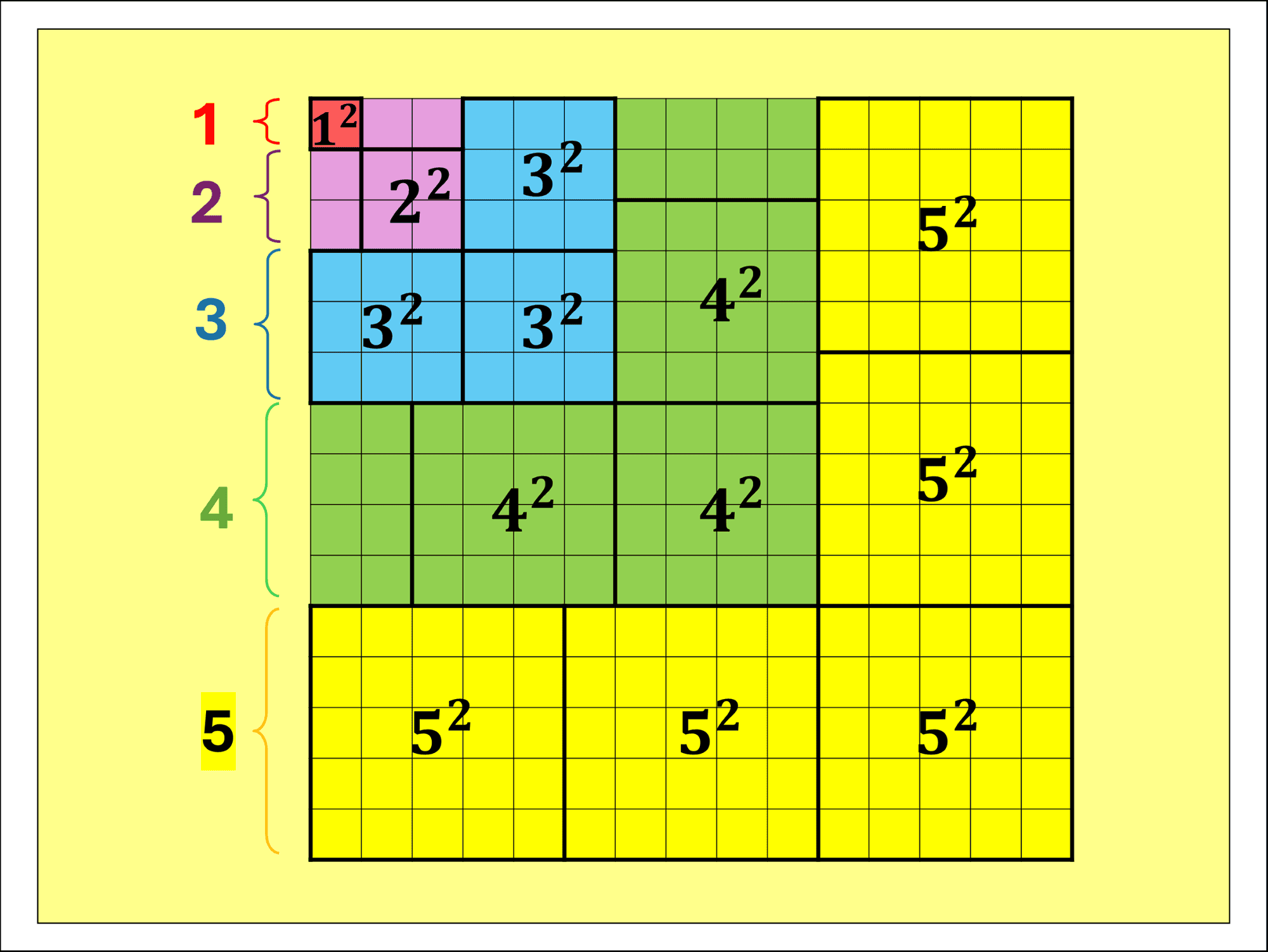
So let’s prove that the square of the nth triangular number equals the sum of the first n squares, using n=5 to demonstrate. For the year $2025$, use the same argument but imagine a larger square, using $9$ colours of little square going all the way up to $9$ instead of $5$.
In this image, let’s count the number of little squares in two different ways:
METHOD 1: the numbers at the left side of the image show that there are $1+2+3+4+5=T_5$ rows. There are also $T_5=1+2+3+4+5$ columns. So the total number of little squares is $(1+2+3+4+5)^2=(T_5)^2$.
METHOD 2: the number of little squares is also red+purple+blue+green+yellow
=$1^2+(2\times2^2) + (3\times3^2) + (4\times4^2)+(5\times5^2)$
$=1^3+2^3+3^3+4^3+5^3$
Note how in the case of odd numbers like 5 (yellow): it’s easy to see that there are five squares each consisting of $5^2$ little squares. But with even numbers like 4 (green): we can see three squares each consisting of $4^2$ little squares, but we have to mentally group the two “half-squares” at the ends together to make the final 4×4 square. So the number of little green squares is $4\times 4^2=4^3$
Because both methods must give the same answer, we have now shown that $(1+2+3+4+5)^2=1^3+2^3+3^3+4^3+5^3$.
You can probably see how we could prove a similar result going all the way up to 10 instead of 5 by just using a larger square with more colours.
A YEAR TO LOOK FORWARD TO: You can bet that in the year 3025, all us mathematicians will be excited that 3025 is both the square of the sum of the first ten whole numbers, and the sum of the first ten cube numbers! If you’re planning a long life – this is definitely worth sticking around to witness!