Thinking of taking A-level maths? Here are some key differences you’ll find from the style of GCSE maths, all designed to make our life easier not harder:
1) EMBRACE THE ALGEBRA!
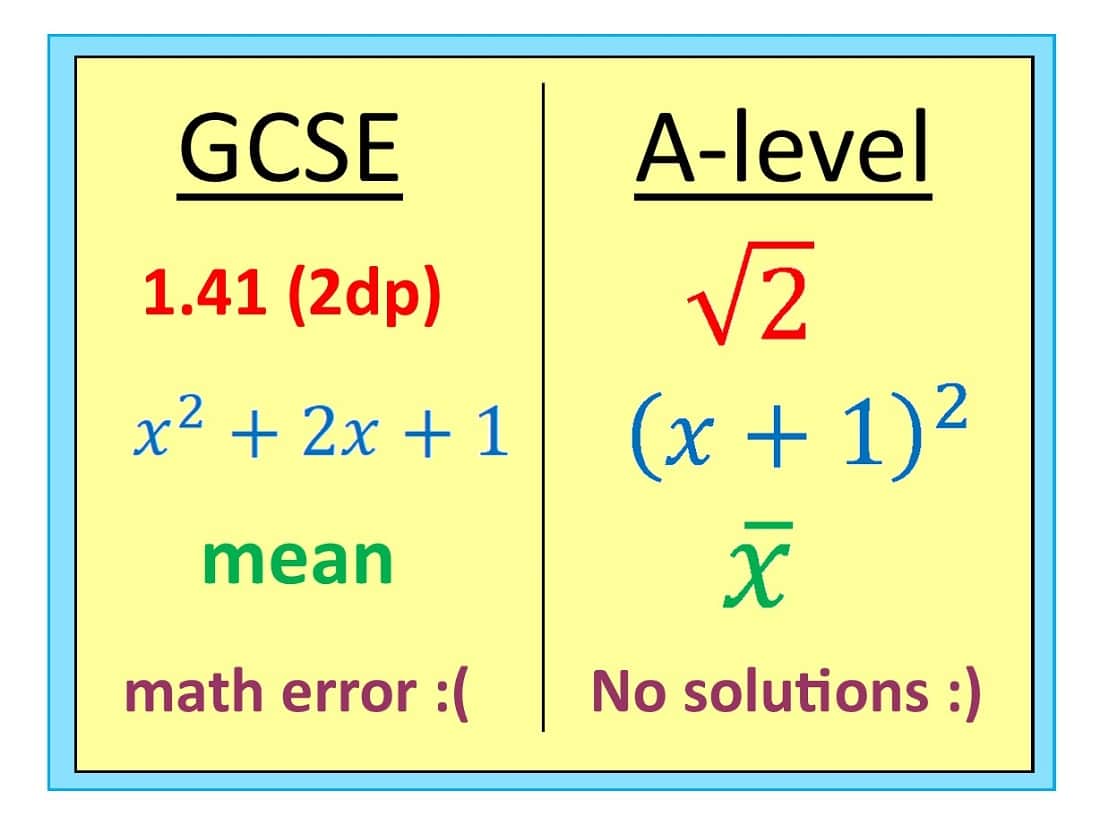
Maths is ultimately about spotting patterns, and algebra is the language we use to write down patterns. A simple example: $A=l \times w$ is a formula to tell you how big a rectangle is. ANY rectangle. At GCSE, it is reasonable to develop clever tools to avoid having to use algebra; but at A-level, we must embrace algebra: it’s our friend and is there to make maths easier! An example: at GCSE the mean is “sum of values divided by number of values” whereas at A-level, the mean gets its own symbol and formula:
$\large{\bar{x}=\frac{\Sigma x}{n}}$
2) LEARN TO LOVE TOP HEAVY FRACTIONS!
Which is better: $1.5$, $\large{1} \frac{1}{2}$ or $\frac{3}{2}$?
At GCSE, many students prefer decimals to fractions. If you have to have fractions, we would certainy convert any top heavy fractions to mixed numbers e.g. $\large { \frac{3}{2}} = \large 1 \frac{1}{2}$. But at A-level, we not only prefer fractions to decimals, but we actually prefer the top-heavy fraction to the mixed number. Why on earth is this? Quite simply: it makes multiplication and division easier. Take $1.5 \times 2.75$. Not so easy: but switch to top-heavy fractions and we just multiply tops and bottoms: $\large {\frac{3}{2} \times \frac{11}{4} = \frac{33}{8}}$
3) FACTORISE!
At GCSE, the first thing to do when seeing brackets is to expand (remove) them: we multiply out the brackets. But at A-level we take the opposite approach: stick those x’s back into brackets! Why? Because when expressions are factorised it avoids us having to write repeated terms out more than once; and if an algebraic fraction is factorised, you can easily spot any possible cancelling.
4) DON’T BE RULED BY YOUR RULER!
In fact, you won’t even need a ruler at A-level maths, unless it’s to divide your page into columns. This is because we now sketch rather than draw diagrams: it’s just the general shape we’re after, not a scale drawing.
5) USE A CALCULATOR:
So you want to solve a quadratic equation? At GCSE you might use the quadratic formula. At A-level you can just type it into the calculator. There’s no non-calculator paper at A-level: so go ahead and use it to evaluate tedious arithmetic. By the way, you will need a more advanced calculator than the standard GCSE one: something like the Casio fx-991. And still make sure you can do simple sums in your head to speed up the easy stuff.
6) MATHS ERROR? NO PROBLEM!
At GCSE, getting a MATH ERROR probably means you have done something wrong. But at A-level, a Math Error might simply mean that your equation has no solutions. So maybe that line doesn’t cut that curve at all, or maybe your population of squirrels will never go extinct, or maybe that bath will never overflow (yes we relate maths to real-world problems – it can be useful!).
7) EXACT FORM:
At GCSE we tend to round our answers to 1 or 2 decimal places. At A-level we more or less abandon decimal places, preferring the more professional “three significant figures” (3sf ensures you’ll be using the same degree of accuracy even if you switch from say kilograms to grams) or better still just leaving the answer in exact form, such as $\sqrt{2}$ or $2\pi$ or $ln(2)$ or $\frac{1}{e}$. By the way, the number e is also new at A-level; like π this is a number that occurs in nature, it’s a little harder than π to see why it occurs in nature but also stunningly elegant when in the second year of A-level we find out why. This and many more treats await you in A-level maths!